IN TUNINGLAND ! (My new Just Int.)

To read about this tuning in its right place, click HERE
And in the video you can hear a version of my tuning with 36 tones pr. octave :

IN TUNINGLAND

After a lot of work with my website TU you – a change to TU and living with the imperfections of these tunings, it became natural for me to move on working with tunings with more than 12 pitches per octave. "In Tuningland" is a result of these efforts.

In Tuningland (IT) is a new idea (presumably) to tune and arrange ordinary keyboards etc. IT operates in the fields of "Just Intonation" with pure or almost pure chords and hides the problem of the comma to quite a large extent.

And my main goal has been that IT can be useful in some way on acoustic instruments.

Along the way in this work, my assessment of the application has shifted between consider IT as just a funny curiosity, and towards that IT can actually have a musical significance.

The last part of this long process resulted in recordings with 2 acoustic harpsichords and two musicians.

After this last experiment IT is now upgraded and confirmed in my mind to at least "social and musical fun in the rehearsal room" !

Since it is easier to play this tuning with 2 or more musicians, let me therefore call it a social tuning. :)

After the acoustic tests and recordings, I also found that I would give harpsichord players an opportunity to test this without having to go too much into the theory.

So this shortcut is for you: first an untempered (not my favorite) version of IT with easy tunings instructions and audio files from real harpsichords.
In the bottom of the page you will see a link to tuning instructions for In Tuningland in tempered versions.
If you've ever longed to play pure chords on your harpsichord , you'll get exclusively pure chords from Ab inclusive E (seen from the circle of fifths).

Click HERE

Another acoustic instrument I've had in mind is the accordion.

This instrument is like the harpsichord rich in overtones and the difference between tunings becomes significant. Unfortunately, the accordion is not as easy to tune as a harpsichord..... If you will anticipate hearing my digital recordings you can find them by scrolling down HERE.

And finally a piece for digital church organ with comparings between 3 variants of In Tuningland + 1/4 and 1/6 comma meantone and 12-ET (Equal temperament)
Click HERE !

If you experience alternative tunings strange, it might be a blend of the fact that 12-ET is ubiquitous in our world and therefore feels unaccustomed, together with the syntonic comma as a problem that still can be present in different subtle ways when working with pure intervals.

I will recommend this introduction to "In Tuningland" also to those who are more interested in the problem of the syntonic comma than in this spesific tuning. This comma is present in all traditional Western music, and not only for the keyboard players. This website has a lot of audio files combined with understandable lattices that provide insight into these issues.

These terms will be explained, visualized and "audiolized" :

1. Comma shift

2. Comma drift

3. Wolf intervals

These phenomena are problems but actually also solutions.

In addition, there are many comparisions with traditional temperaments (Meantones (1/4&1/6,Equal T.) where you can judge by yourself and train your ear at the same time.

.......

For those familiar with the terminology you can click below on the table of contents for a quick survey :

1. Arranging of the keyboard
2. Supplementary and overlapping setup
3. Halving the comma shift
4. Economizing amount of pitches
5. Small adjustments (tempering)
6. Expansions
7. Comprehensive setups

And here are the pluses and minuses with IT (click)

......................................................................................................................

In this guide to "In Tuningland", I will like to use completely pure intervals and chords.

Then what is right and what is wrong will be very clear.

Due to an extensive use of audio files combined with lattices, I can postpone the use of cent values and the calculation until the end of this introduction.

The fascination of tuning and the frustration that the world of fifths and world of thirds doesn`t fit together have haunted musicians for centuries. Common to all endeavors lies in dealing with commas, not least the syntonic comma.

The syntonic comma is the most important comma, in any case in traditional western music.

This comma is markedly present when string players intonate. (The Pythagorean comma, which is maybe more often mentioned, refers to tuning of keyboard instruments and to solo instruments more indirectly)

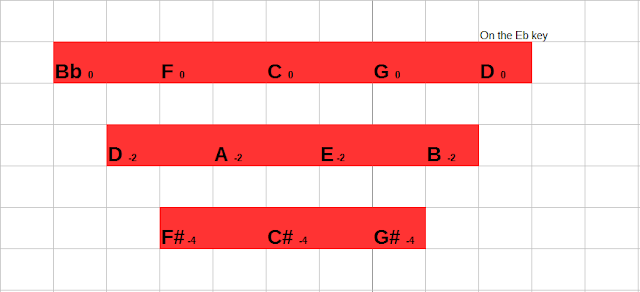
The fact is: if we tune 4 pure fifths one after another, such as Bb-F-C-G-D, then this D, named by me as "D 0", is annoying higher than the one we get when tuning a pure major third from the same starting point, Bb-D (named "D -2").
The difference between "D 0" and "D-2" is exactly one syntonic comma (in this untempered version)

The reason why I define the difference as two units (D 0 and D -2) is that I will be completely consistent in my explanations to avoid confusions.

Later we want to use 1 unit , a half of a comma.

1. Arranging of the keyboard

My starting point is pure thirds and fifths on a regular keyboard in C major.
This C-setup is marked C

In C, pure C major, F major, Dm, Am, Em, G major, Bb major, D major, A major, E major are prioritized.

For this we need 2 D`s, where the highest is placed on the Eb key !

With this setup, we get three pure fifth rows :

Before this is getting too confusing, we will hurry to our first listening example.

Such a tuning contains many chords, but because of the problem with the comma, it also has big limitations, so it is not usable for so much music.

I will nevertheless demonstrate all the 3-tone major and minor triads in a small piece where I've steered away from the comma problem, but the curse of the comma will appear in the end. : (

......................

Video (music piece) : C 0 Passing through all pure 3-tones triads + many more

..............................

Important:

The observant listener will see and hear that:

Pure major thirds are obtained from the fifth row below (- 2 units).

Pure minor thirds are obtained from the fifth row above(+ 2 units)..

..........

A pure sus4 chord is 3 tones (2 fifths) horizontally in a row.

Furthermore, we see that a pure D sus4 in this first example, C, is missing (No G-D-A in a horizontal line).

Around G-D-A there are major problems, we have a pure G-(high)D and a pure (low)D-A , but with 2 different D`s, not successive.

Pure intervals will easily make some other wolf intervals.
The intervals between the red tones below are wolfs, they are not neighbors on a row in the diagram.

But if we avoid them we have actually 3 similar diatonic scales
First C major
C-D(on Eb key)- E- F- G- A-B- C

If we only use one of the red tones at a time (avoid the wolf fifth (high)D-A) , we will get a very pure feeling with cluster possibilities.
The tritonus is as pure as possible.
Notice that the minor third D-F is not pure , but can be used.

Then we have F major.
F-G-A-Bb-C-D(the lower one)E-F

Only use one red tone at a time (avoid the wolf G-(low)D). G-Bb is not pure.

The nice thing is that on the other side of the Dsus4 division (from the view of circle of fifths) we will find exactly the same possibilities in A major (do not play B and F# at the same time).
Only use one red tone at a time.

A-B-C#-D(the lower one)-E-F#-G#-A

To be familiar with this setup in C, it is very useful to play these scales and chords.
Also highly recommended is to play 2 melodic minor scales with their associated chords.
First in A minor.

Don`t use B and F# at the same time Avoid ii -chord

A-B-C-D-E-F#-G#-A

So D minor, avoid G-D at the same time .
Avoid IV -chord

Here we can play I-ii-V-I (D(m)-Em-A-D(m))

D-E-F-G-A-B-C#-D

If you have tried scales and chords in this setup, you are ready for a transposing to Bb
............................................

2. Supplementary and overlapping setup

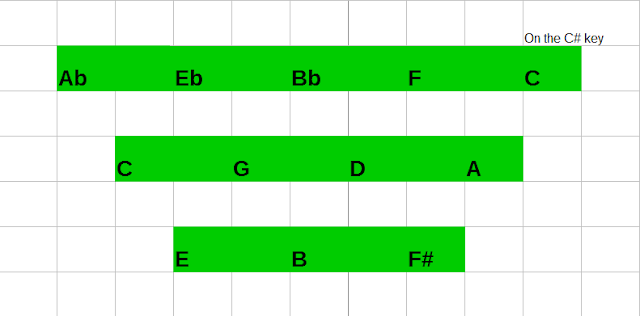
If we transpose this tuning to Bb we get 2 C`s (instead of 2 D`s), the highest of them is placed on the C# key. .

Video (music piece) : Bb +1 Passing through all pure 3-tones triads + many more

Now we see that we have both a pure Dsus4 (G-D-A) and the opportunity to play a pure Dm-G (not 7th) -C progression. The problem has been moved to Csus4. F-C-G
The text about scales and chords from C 0 above , are transposed to Bb HERE !

But couldn`t we just let Bb take over when we meet problems in C ??
Here I have merged them with a common area in grey.

Like this:

But then we get a new big problem. We end up somewhere else than where we started, a phenomenon called comma drift.

Hear and see this in extreme, only pure chords, and the common tones between the chords are the same.
This is the syntonic comma at its worst and funniest :)

Video: RISING !

Video: FALLING !

Bb, is resolving something by giving other chords and scales, but we still have the urgent comma problem.

.....................................

3. Halving the comma shift

Here we are in the core of "In Tuningland".

We now raise Bb by half of a comma, to a Bb +1.
Like this :

The same displayed in another way :

Then we will get a D in Bb +1, now denoted as D -1 that will end in the middle of D 0 and D-2 in C 0.

The comma shift is halved while these two manuals complement each other chordally.

And if we go to the problem with the two C's in Bb + 1 which after the rising gives them the names C +1 and C -1,then we see that C 0 from C 0 is lying in the middle of these two tones in the same way.

If we are to sum up this IT variant with the lowest amount of pitches (24), which can be tuned on a two-manual harpsichord, then we now can see that these manuals are overlapping and complementing each other in a nice way.

1. Some chords are only available just on one manual.

Some chords are to be found on both manuals.

2. Some chords are pure just on one manual.

Some chords are pure on both manuals.

3. You can change the manual when the curse of the comma threatens.

In a way, we have a comma shift and a comma drift at the same time, but only the half of it.

Now we have complementary setups with a halved comma shift.

A nice thing : We have Dm, Am, Em,......, F#m , C#m , but miss Bm in C 0

But we do have Bm in Bb+1 (which is a more distant key) !

In the two first examples I am dancing with the wolfes :) :

C 0 with the comma problem : Video HERE

Bb+1 with the comma problem : Video HERE

.................................................................

C0 and Bb+1 combined, and the comma problem reduced: Video:HERE

The chords from C 0 and Bb +1 are interlaced into each other.

Some chords have a bit higher pitch in Bb +1 than in C 0 and vice verca.

A survey for the chords is available HERE.

I started with C 0 because it is easiest to understand and I have taken a starting point in this. However, if we look at the range in scales and chords, it is maybe more natural selecting F 0 and G-1 to get C major as the center.

Here is a view of the scales (not chords):

Bb +1 C 0	F 0 G -1
Eb major	Bb major
Bb major	F major
F major	C major
C major	G major
G major	D major
A major	E major

C minor	G minor
G minor	D minor
D minor	A minor
A minor	E minor

4. Economizing amount of pitches

This is an economizing model and can not be compared with the digital world's approach to infinite pitch adjustments.

Working with Just Intonation on digital instruments:

you can take a look on this APP,

and this SOFTWARE.

But it is much more difficult to find a tuning that is economizing regarding amount of pitches and opens up for using it in a acoustic way.

Because of the relative few pitches IT can be used on one or several double manual harpsichords. Just Intonation is really something different playing it acoustically.

"Yes, many find the purity of computer-generated sounds utterly unnatural. The reason is that there will be a fixed pattern of phase cancellation among some of the coinciding partials depending on your onset times. This frozen pattern of phase cancellation does not occur in real music, where phase relationships among coinciding partials will cycle between constructive and destructive. Without this effect, the dyad or chord will sound like a single timbre rather than a harmony." Paul Erlich

Of course you can (like me) use In Tuningland in a digital way, if your keyboard/software allows IT.

5. Small adjustments (tempering)

Until now we have operated exclusively with pure intervals.
Those who will try to tune their double-manual harpsichord can use this table. Or you can tune by ear using TUNING INSTRUCTIONS
You can do it on a digital keyboard too, but if the two pitches of D in C 0 and two Cs in Bb+1 make troubles then it will be better using the spreadsheets. Explanation below.

If the comma shift still is annoying then my solution and maybe my favourite version is to narrow/temper all fifths with 1 cent. Then the comma shift will decrease from 10,8 to 8,8 cent.
Here is the table for this.

You can use the spreadsheet to find your own solution. See further down.
The tempered version is used both in
15 HYMNS,
the acoustic harpsichord recordings and
2 simple improvisations.
My (digital) accordion recordings with comparing possibilities
can be heard HERE. (Read or scroll down)

There are pluses and minuses when chosing the quality of the fifths.

Take a look : HERE

Here is a collection to get familiar with In Tuningland in the smallest version (24 pitches). It is 15 hymns with lattices and I have also lowered the speed. In slow tempo you get an opportunity to follow the idea and is my best examples to explain the idea.

You can also choose to compare IT with 1/4 comma meantone and Equal temperament.

VIDEOS, 15 Hymns : HERE

6. Expansions

The principle of "In Tuningland" can be expanded.

By replacing only 5 tones in C 0 we get exactly the corresponding setup transposed to Ab,

marked as Ab +2.
In the same way as in C 0 and Bb +1 we have two pitches of a tone, in Ab +2 we have Bb:
Bb 0 and a Bb +2, the latter on the B key.

Video: Here are chords in Ab +2

And if we replace 5 tones in Bb + 1 we can get D -1.
Here we have two pitches of E :
E-3 and E-1, the last placed on the F key.

Video: Here are the chords in D -1

Tables of the chords available , where to find and which is highest in pitch: HERE

Below you will find the notes interlaced.
The darkest red is common for Ab+2 and C 0
The darkest green is common for Bb+1 and D-1.
The five tones in lilac on the top belongs to Ab+2.
The five light red to the right belongs to C 0.
The five lighter green notes in 2nd row belongs to Bb+1.
The five light green notes to the right belongs to D-1.

IT provides here a wide range of chords using 34 pitches.

In this piece I will show some of the possibillities. Everytime I shift keyboard/setup there will come a sign up to the left.
And you can compare it with common temperaments.
In meantone (1/4&1/6) only 8 major thirds are useful.
In my comparisions I use extended meantone, that means
more than 12 pitches pr.octave, similar to keyboards with split keys . Here my focus is on the qualtites of the sound and not the practical solutions for the meantones.

	Full tempo	1/2 tempo	1/3 tempo
IT Pure fifths	VIDEO	VIDEO	VIDEO
IT -1 cent fifths	VIDEO	VIDEO
IT -2 cent fifths	VIDEO	VIDEO
1/4 c meantone	VIDEO	VIDEO
1/6 c meantone	VIDEO	VIDEO
Equal temperament	VIDEO	VIDEO

Here it must be admitted that when we use the version of -1 cent tempered fifths, my favorite, and the sound has a little less treble and it is a bit fuller, so the difference to the meantone(1/4) is not so great as expected. But you can hear it.

Here is the right time for you to judge by yourself and train your ears using my little organ piece
"A PURE MIX"

..............................

Of course, economic use of the number of pitches is not so important in the advanced part of the digital tuning world, and even with two acoustic harpsichords we are still using 48 keys on these 34 pitches.
But used on an archicembalo this will be of utmost importance.
(See spreadsheet link to archicembalo down below.)
It would be nice to test In Tuningland on a harpsichord or organ
with Vicentino`s keyboard idea !

https://en.wikipedia.org/wiki/Archicembalo

And if IT is used on an organ (like the Groven organ) where the right pitch is chosen automatic, the advantage of few pitches is obvious.

7. Comprehensive setups

Under point 6. the number of chords was expanded using 4 setups: Ab +2, Bb +1, C 0 and D -1.

Apart from the very nice growth of chords, flexibility does not get so much better.

In the beginning of this introduction I was promoting a nice version for 2 harpsichords .

We can use Bb +1, F 0, C 0 and G -1 (32 pitches per octave).

This system provides much better handling of passing tones and will allow access to some material from the renaissance. The advantage of 2 cembalists is that each of them has only 2 tones on the wrong keys each to keep track of. And with 2 players we will get much more smooth transitions regarding switching of the manuals. See the acoustic and practical page to this idea. HERE !

HERE you can check how many tones that is common in a couple of setups with same color.

This combination provides 2 additional major triads compared to meantone.

We can play pure Ab, B and G#m. Actually we can also play a pure Fm with a combination of 2 manuals (F-C on G-1 and Ab on Bb +1)

Tables of the chords available , where to find and which is highest in pitch:

HERE

The dark red is common for C 0 and F 0,

The dark green is common to Bb +1 and G -1

The lilac to the right is only for C 0,

The lilac to the left only for F 0

The light green on the top is only for Bb +1,

The yellowish green to the left is only for for G -1.

......................................................................................................

Then to the :

SPREADSHEETS

They are made in LibreOffice (It is free to download)
But you can open them in Excel too.

The simplest spreadsheet: C 0 and Bb +1 (Click and download)

Under the green INPUTs you will find the cells where you can enter a variable.
The green INPUT at the top to the right is just a calculator where you can have comma shift as a variable.
The calculator has no effect on the main tables.
Up to the left you will find:

I always let the major third be pure (0) , but if you want another value you can change it.

The cell for the fifths is important. If you enter -1 (my favourite) in the Fifth cell, you will see a double reduction (-2 cent) of the comma shift from 10,753 to 8,753.

Then you have 3 INPUTs to the left around C0

If the yellow and blue cells are set to 0 and you enter the values for a setup into a synthesizer or a tuning app, than the tones with a square will be a semitone too high.
Therefore set the blue cell to - 100 to lower these tones with a semitone.
But then these values will get a very low value.
Many synthesizers don`t allow values outside -63/+63.
To fix that enter a positive value into the yellow cell
eg +50. Then all the values will come inside this range.
Another BUT:
But then the cent values will be too high and your desired concert pitch (eg 440 Hz) will be far from right.
So therefore enter eg 440 into the brown cell and just to the right in the light brown cell you will get a Hz value to enter to get the right pitch.
Now everything is fine and you can enter cent numbers for the different setups, in our example C0 with one extra D, and Bb+1 with one extra C, both in squares.
....................................
If you want to delete a setup from the selection in a spreadsheet, then highlight the rows in the margin to the left.
A right click, and delete !